 English
English
Guernica. The Art Gallery Problem: Watch and Enlighten, 2014
In 1973 the mathematician Victor Klee posed the following question: How many guards are necessary, and how many are sufficient, to patrol the paintings and works of art in an art gallery with n walls? This question of combinatorial geometry came to concern scientists in the following decades and is the topic of numerous papers. The six drawings in this installation are speculative examples of the “Art Gallery Problem,” as the question has come to be known. Besides its obvious reference to the theme of visibility, witnessing, and (the impossibility of ) having an overview in Guernica, the drawings also suggest that the logic and rationales informing the contemporary surveillance society have invaded the conception of the gallery space. Not just the artworks but those who look at the artworks are being watched. In other words, The Art Gallery Problem: Watch and Enlighten reframes Klee’s intriguing and seemingly innocent mathematical question as a question of contested politics.
[The art gallery problem or museum problem is a well-studied visibility problem in computational geometry. It originates from a real-world problem of guarding an art gallery with the minimum number of guards who together can observe the whole gallery. In the computational geometry version of the problem the layout of the art gallery is represented by a simple polygon and each guard is represented by a point in the polygon. A set 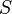 of points is said to guard a polygon if, for every point
of points is said to guard a polygon if, for every point  in the polygon, there is some
in the polygon, there is some 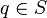 such that the line segment between
such that the line segment between  and
and  does not leave the polygon.]
does not leave the polygon.]

